Abstract data structures

Abstract data types

In computer science, an abstract data type (ADT) is a mathematical model for data types where a data type is defined by its behavior (semantics) from the point of view of a user of the data, specifically in terms of possible values, possible operations on data of this type, and the behavior of these operations. This contrasts with data structures, which are concrete representations of data, and are the point of view of an implementer, not a user.

Formally, an ADT may be defined as a "class of objects whose logical behavior is defined by a set of values and a set of operations"; this is analogous to an algebraic structure in mathematics. What is meant by "behavior" varies by author, with the two main types of formal specifications for behavior being axiomatic (algebraic) specification and an abstract model; these correspond to axiomatic semantics and operational semantics of an abstract machine, respectively. Some authors also include the computational complexity ("cost"), both in terms of time (for computing operations) and space (for representing values).

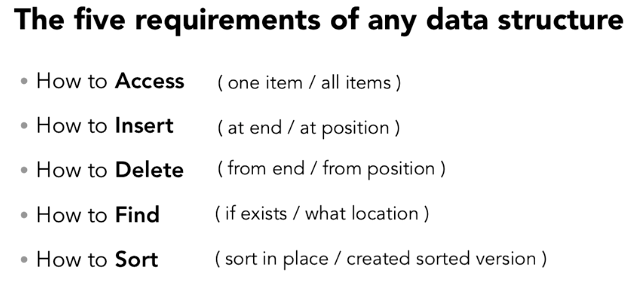
Basic operations of data structures

The big ideas in abstract data structures

Thinking recursively

• Recursive thinking
• Recursive algorithms

Abstract data structures

• Two dimensional arrays
• Stack
• Queue

Linked lists

• Dynamic data structures
• Linked lists

Trees

• Trees

Standards

• Identify a situation that requires the use of recursive thinking.
• Identify recursive thinking in a specified problem solution.
• Trace a recursive algorithm to express a solution to a problem.

• Describe the characteristics of a two- dimensional array.
• Construct algorithms using two- dimensional arrays.
• Describe the characteristics and applications of a stack.

• Construct algorithms using the access methods of a stack.
• Describe the characteristics and applications of a queue.
• Construct algorithms using the access methods of a queue.
• Explain the use of arrays as static stacks and queues.
• Describe the features and characteristics of a dynamic data structure.
• Describe how linked lists operate logically.

• Sketch linked lists (single, double and circular).
• Describe how trees operate logically (both binary and non-binary).
• Define the terms: parent, left-child, right-child, subtree, root and leaf.
• State the result of inorder, postorder and preorder tree traversal.
• Sketch binary trees.
• Define the term dynamic data structure.
• Compare the use of static and dynamic data structures.
• Suggest a suitable structure for a given situation.

Provide an answer from a number of possibilities. Recognize and state briefly a distinguishing fact or feature.

Follow and record the action of an algorithm.

Give a detailed account or picture of a situation, event, pattern or process.

Develop information in a diagrammatic or logical form.

Give a detailed account including reasons or causes.

Represent by means of a diagram or graph (labelled as appropriate). The sketch should give a general idea of the required shape or relationship, and should include relevant features.

Give the precise meaning of a word, phrase, concept or physical quantity.

Give a specific name, value or other brief answer without explanation or calculation.

Give a specific name, value or other brief answer without explanation or calculation.

Propose a solution, hypothesis or other possible answer.